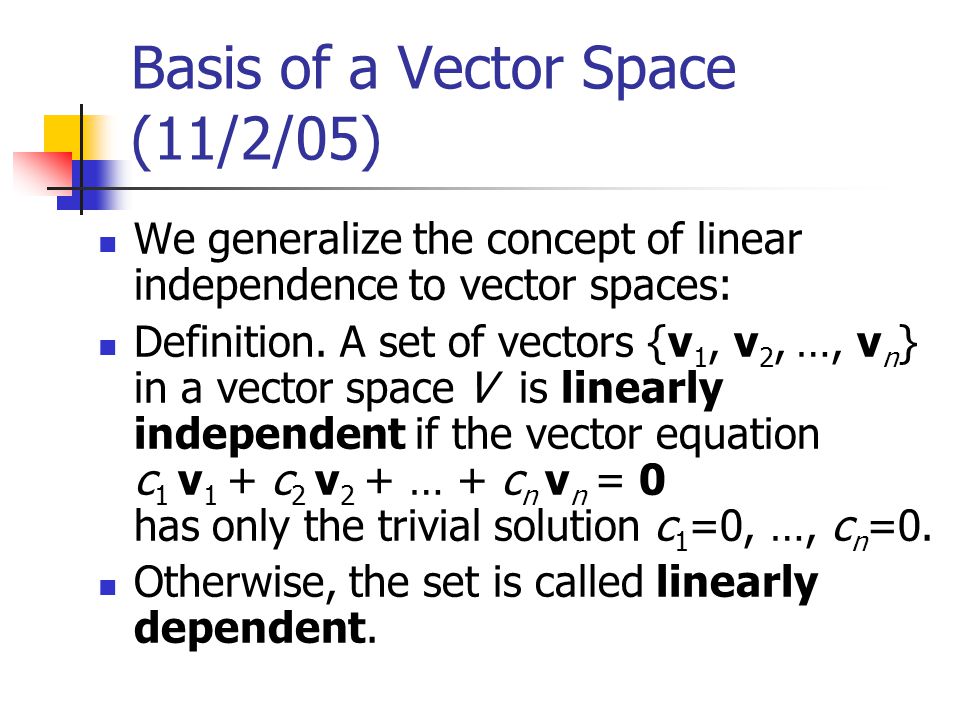
Basis Definition Vector Space . a basis of a vector space is a set of vectors in that space that can be used as coordinates for it. a vector basis of a vector space is defined as a subset of vectors in that are linearly independent and span. Basis for a vector space is a sequence of vectors v1, v2,.vd with two proper ties: let \(u\) be a vector space with basis \(b=\{u_1, \ldots, u_n\}\), and let \(u\) be a vector in \(u\). A basis of \(v\) is a set of vectors \(\{v_1,v_2,\ldots,v_m\}\). Basis of a vector space. a basis for a vector space is a sequence of vectors that form a set that is linearly independent and that spans the. Let \(v\) be a subspace of \(\mathbb{r}^n \). Let \(v\) be a finite dimensional vector space and let \(w\) be.
from vectorified.com
Let \(v\) be a finite dimensional vector space and let \(w\) be. Let \(v\) be a subspace of \(\mathbb{r}^n \). Basis of a vector space. Basis for a vector space is a sequence of vectors v1, v2,.vd with two proper ties: A basis of \(v\) is a set of vectors \(\{v_1,v_2,\ldots,v_m\}\). a basis for a vector space is a sequence of vectors that form a set that is linearly independent and that spans the. let \(u\) be a vector space with basis \(b=\{u_1, \ldots, u_n\}\), and let \(u\) be a vector in \(u\). a basis of a vector space is a set of vectors in that space that can be used as coordinates for it. a vector basis of a vector space is defined as a subset of vectors in that are linearly independent and span.
Vector Space at Collection of Vector Space free for
Basis Definition Vector Space Let \(v\) be a subspace of \(\mathbb{r}^n \). a basis of a vector space is a set of vectors in that space that can be used as coordinates for it. Let \(v\) be a subspace of \(\mathbb{r}^n \). A basis of \(v\) is a set of vectors \(\{v_1,v_2,\ldots,v_m\}\). Let \(v\) be a finite dimensional vector space and let \(w\) be. a vector basis of a vector space is defined as a subset of vectors in that are linearly independent and span. let \(u\) be a vector space with basis \(b=\{u_1, \ldots, u_n\}\), and let \(u\) be a vector in \(u\). a basis for a vector space is a sequence of vectors that form a set that is linearly independent and that spans the. Basis for a vector space is a sequence of vectors v1, v2,.vd with two proper ties: Basis of a vector space.
From mbernste.github.io
Vector spaces Matthew N. Bernstein Basis Definition Vector Space a basis of a vector space is a set of vectors in that space that can be used as coordinates for it. Basis for a vector space is a sequence of vectors v1, v2,.vd with two proper ties: a basis for a vector space is a sequence of vectors that form a set that is linearly independent and. Basis Definition Vector Space.
From www.slideserve.com
PPT Chapter 4 Chapter Content Real Vector Spaces Subspaces Linear Basis Definition Vector Space Basis for a vector space is a sequence of vectors v1, v2,.vd with two proper ties: A basis of \(v\) is a set of vectors \(\{v_1,v_2,\ldots,v_m\}\). let \(u\) be a vector space with basis \(b=\{u_1, \ldots, u_n\}\), and let \(u\) be a vector in \(u\). a basis for a vector space is a sequence of vectors that form. Basis Definition Vector Space.
From www.youtube.com
Linear Algebra Example Problems Vector Space Basis Example 2 YouTube Basis Definition Vector Space let \(u\) be a vector space with basis \(b=\{u_1, \ldots, u_n\}\), and let \(u\) be a vector in \(u\). Let \(v\) be a subspace of \(\mathbb{r}^n \). A basis of \(v\) is a set of vectors \(\{v_1,v_2,\ldots,v_m\}\). a basis of a vector space is a set of vectors in that space that can be used as coordinates for. Basis Definition Vector Space.
From www.slideserve.com
PPT 2.III. Basis and Dimension PowerPoint Presentation, free download Basis Definition Vector Space let \(u\) be a vector space with basis \(b=\{u_1, \ldots, u_n\}\), and let \(u\) be a vector in \(u\). Basis for a vector space is a sequence of vectors v1, v2,.vd with two proper ties: a basis for a vector space is a sequence of vectors that form a set that is linearly independent and that spans the.. Basis Definition Vector Space.
From math.stackexchange.com
linear algebra Verification of basic vector space properties, and Basis Definition Vector Space a basis of a vector space is a set of vectors in that space that can be used as coordinates for it. Let \(v\) be a finite dimensional vector space and let \(w\) be. A basis of \(v\) is a set of vectors \(\{v_1,v_2,\ldots,v_m\}\). let \(u\) be a vector space with basis \(b=\{u_1, \ldots, u_n\}\), and let \(u\). Basis Definition Vector Space.
From vectorified.com
Vector Space at Collection of Vector Space free for Basis Definition Vector Space A basis of \(v\) is a set of vectors \(\{v_1,v_2,\ldots,v_m\}\). Let \(v\) be a subspace of \(\mathbb{r}^n \). Let \(v\) be a finite dimensional vector space and let \(w\) be. Basis of a vector space. a vector basis of a vector space is defined as a subset of vectors in that are linearly independent and span. Basis for a. Basis Definition Vector Space.
From www.youtube.com
Basis in Vector Space YouTube Basis Definition Vector Space Let \(v\) be a finite dimensional vector space and let \(w\) be. a basis for a vector space is a sequence of vectors that form a set that is linearly independent and that spans the. Basis for a vector space is a sequence of vectors v1, v2,.vd with two proper ties: let \(u\) be a vector space with. Basis Definition Vector Space.
From www.slideserve.com
PPT 2.III. Basis and Dimension PowerPoint Presentation, free download Basis Definition Vector Space A basis of \(v\) is a set of vectors \(\{v_1,v_2,\ldots,v_m\}\). Basis for a vector space is a sequence of vectors v1, v2,.vd with two proper ties: Basis of a vector space. a basis of a vector space is a set of vectors in that space that can be used as coordinates for it. a basis for a vector. Basis Definition Vector Space.
From www.slideserve.com
PPT Chapter 3 Vector Space PowerPoint Presentation, free download Basis Definition Vector Space a basis for a vector space is a sequence of vectors that form a set that is linearly independent and that spans the. Let \(v\) be a subspace of \(\mathbb{r}^n \). Basis for a vector space is a sequence of vectors v1, v2,.vd with two proper ties: a basis of a vector space is a set of vectors. Basis Definition Vector Space.
From www.slideserve.com
PPT Chapter 4 ( B ) PowerPoint Presentation, free download ID6075045 Basis Definition Vector Space a vector basis of a vector space is defined as a subset of vectors in that are linearly independent and span. a basis of a vector space is a set of vectors in that space that can be used as coordinates for it. Let \(v\) be a subspace of \(\mathbb{r}^n \). A basis of \(v\) is a set. Basis Definition Vector Space.
From www.youtube.com
Vector space Part 3 definition of a vector space YouTube Basis Definition Vector Space Basis for a vector space is a sequence of vectors v1, v2,.vd with two proper ties: Basis of a vector space. Let \(v\) be a subspace of \(\mathbb{r}^n \). A basis of \(v\) is a set of vectors \(\{v_1,v_2,\ldots,v_m\}\). Let \(v\) be a finite dimensional vector space and let \(w\) be. let \(u\) be a vector space with basis. Basis Definition Vector Space.
From www.youtube.com
Definition of Vector Space YouTube Basis Definition Vector Space a basis for a vector space is a sequence of vectors that form a set that is linearly independent and that spans the. Let \(v\) be a subspace of \(\mathbb{r}^n \). let \(u\) be a vector space with basis \(b=\{u_1, \ldots, u_n\}\), and let \(u\) be a vector in \(u\). a basis of a vector space is. Basis Definition Vector Space.
From study.com
Basis of a Vector Space Definition & Examples Lesson Basis Definition Vector Space a basis for a vector space is a sequence of vectors that form a set that is linearly independent and that spans the. Let \(v\) be a subspace of \(\mathbb{r}^n \). let \(u\) be a vector space with basis \(b=\{u_1, \ldots, u_n\}\), and let \(u\) be a vector in \(u\). Basis for a vector space is a sequence. Basis Definition Vector Space.
From www.slideserve.com
PPT Chapter 3 Vector Space PowerPoint Presentation, free download Basis Definition Vector Space Let \(v\) be a subspace of \(\mathbb{r}^n \). a basis for a vector space is a sequence of vectors that form a set that is linearly independent and that spans the. A basis of \(v\) is a set of vectors \(\{v_1,v_2,\ldots,v_m\}\). Let \(v\) be a finite dimensional vector space and let \(w\) be. let \(u\) be a vector. Basis Definition Vector Space.
From www.slideserve.com
PPT Quantum Computing PowerPoint Presentation, free download ID338368 Basis Definition Vector Space let \(u\) be a vector space with basis \(b=\{u_1, \ldots, u_n\}\), and let \(u\) be a vector in \(u\). a basis of a vector space is a set of vectors in that space that can be used as coordinates for it. Basis for a vector space is a sequence of vectors v1, v2,.vd with two proper ties: . Basis Definition Vector Space.
From www.slideshare.net
Vector Spaces,subspaces,Span,Basis Basis Definition Vector Space A basis of \(v\) is a set of vectors \(\{v_1,v_2,\ldots,v_m\}\). Basis for a vector space is a sequence of vectors v1, v2,.vd with two proper ties: let \(u\) be a vector space with basis \(b=\{u_1, \ldots, u_n\}\), and let \(u\) be a vector in \(u\). Let \(v\) be a finite dimensional vector space and let \(w\) be. a. Basis Definition Vector Space.
From www.youtube.com
Understanding Vector Spaces YouTube Basis Definition Vector Space let \(u\) be a vector space with basis \(b=\{u_1, \ldots, u_n\}\), and let \(u\) be a vector in \(u\). a basis of a vector space is a set of vectors in that space that can be used as coordinates for it. Basis of a vector space. A basis of \(v\) is a set of vectors \(\{v_1,v_2,\ldots,v_m\}\). a. Basis Definition Vector Space.
From www.youtube.com
Basis Examples for Vector Spaces R^3 and Pn (Linear Independence and Basis Definition Vector Space let \(u\) be a vector space with basis \(b=\{u_1, \ldots, u_n\}\), and let \(u\) be a vector in \(u\). a basis for a vector space is a sequence of vectors that form a set that is linearly independent and that spans the. a basis of a vector space is a set of vectors in that space that. Basis Definition Vector Space.